Are fast scramblers good thermal baths?

Abstract

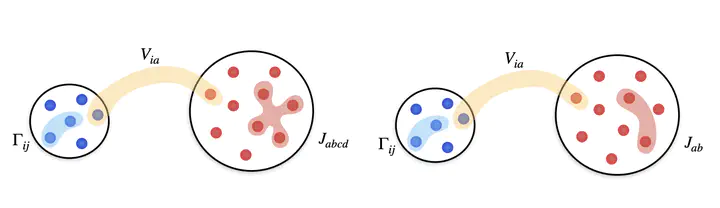
The Sachdev-Ye-Kitaev (SYK4) model has attracted attention for its fast scrambling properties and its thermalization rate that is set only by the temperature. In this work we ask the question of whether the SYK4 model is also a good thermal bath, in the sense that it allows a system coupled to it to thermalize. We address this question by considering the dynamics of a system of N random non-interacting Majorana fermions coupled to an SYK4 bath with M Majorana fermions that we solve with Keldysh techniques in the limit of M≫N≫1. We compare this nonequilibrium setting with a conventional bath made of free non-interacting degrees of freedom with a continous spectrum. We show that the SYK4 bath is more efficient in thermalising the system at weak coupling, due to its enhanced density of states at low frequency, while at strong system-bath couplings both type of environments give rise to a similar time scale for thermalisation.